The parallax is mostly used to determine distances in nearby space. The parallax is the angular difference between two measurements of the position of the object from different view-points. If the annual parallax is given by p, the distance R of the object is given by R=a/sin(p), in which a is the radius of the Earth’s orbit. The clusterparallax is used to determine the distance of a group of stars by using their motion w.r.t. a fixed background. The tangential velocity vt and the radial velocity vr of the stars along the sky are given by
vr=Vcos(θ) , vt=Vsin(θ)=ωR
where θ is the angle between the star and the point of convergence and ˆR the distance in pc. This results, with vt=vrtan(θ), in:
R=vrtan(θ)ω ⇒ ˆR=1″ where p is the parallax in arc seconds. The parallax is then given by p=\frac{4.74\mu}{v_{\rm r}\tan(\theta)}

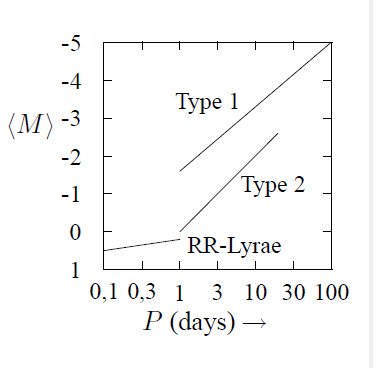
with \mu the proper motion of the star in ''/yr. A method to determine the distance of objects which are somewhat further away, like galaxies and star clusters, uses the period-Brightness relation for Cepheids. This relation is shown in the above figure for different types of stars.
The brightness is the total radiated energy per unit of time. Earth receives s_0=1.374 kW/m^2 from the Sun. Hence, the brightness of the Sun is given by L_\odot=4\pi r^2s_0=3.82\cdot10^{26} W. It is also given by:
L_\odot=4\pi R_\odot^2\int\limits_0^\infty \pi F_\nu d\nu
where \pi F_\nu is the monochromatic radiation flux. At the position of an observer this is \pi f_\nu, with f_\nu=(R/r)^2F_\nu if absorption is ignored. If A_\nu is the fraction of the flux which reaches Earth’s surface, the transmission factor is given by R_\nu and the surface of the detector is given by \pi a^2, then the apparent brightness b is given by:
b=\pi a^2\int\limits_0^\infty f_\nu A_\nu R_\nu d\nu
The magnitude m is defined by:
\frac{b_1}{b_2}=(100)^{\frac{1}{5}(m_2-m_1)}=(2.512)^{m_2-m_1}
because the human eye perceives light intensities logaritmicaly. From this follows that m_2-m_1=2.5\cdot^{10}\log(b_1/b_2), or: m=-2.5\cdot^{10}\log(b)+C. The apparent brightness of a star if this star would be at a distance of 10 pc is called the absolute brightness B: B/b=(\hat{r}/10)^2. The absolute magnitude is then given by M=-2.5\cdot^{10}\log(B)+C, or: M=5+m-5\cdot^{10}\log(\hat{r}). When an interstellar absorption of 10^{-4}/pc is taken into account one finds:
M=(m-4\cdot10^{-4}\hat{r})+5-5\cdot^{10}\log(\hat{r})
If a detector detects all radiation emitted by a source one would measure the absolute bolometric magnitude. If the bolometric correction BC is given by
BC=2.5\cdot^{10}\log\left(\frac{\mbox{Energy flux received}}{\mbox{Energy flux detected}}\right)= 2.5\cdot^{10}\log\left(\frac{\int f_\nu d\nu}{\int f_\nu A_\nu R_\nu d\nu}\right)
then: M_b=M_V-BC where M_V is the visual magnitude. Further M_b=-2.5\cdot^{10}\log\left(\frac{L}{L_\odot}\right)+4.72
The radiation energy passing through a surface dA is dE=I_\nu(\theta,\varphi)\cos(\theta)d\nu d\Omega dAdt, where I_\mu is the monochromatical intensity Wm^{-2} sr^{-1} Hz^{-1} ].
When there is no absorption the quantity I_\nu is independent of the distance to the source. Planck’s law holds for a black body:
I_\nu(T)\equiv B_\nu(T)=\frac{c}{4\pi}w_\nu(T)=\frac{2h\nu^3}{c^2}\frac{1}{\exp(h\nu/kT)-1}
The radiation transport through a layer can then be written as: \frac{dI_\nu}{ds}=-I_\nu\kappa_\nu+j_\nu Here, j_\nu is the coefficient of emission and \kappa_\nu the coefficient of absorption. \int ds is the thickness of the layer. The optical thickness \tau_\nu of the layer is given by \tau_\nu=\int\kappa_\nu ds. The layer is optically thin if \tau_\nu\ll1, the layer is optically thick if \tau_\nu\gg1. For a stellar atmosphere in LTE: j_\nu=\kappa_\nu B_\nu(T). Then also :
I_\nu(s)=I_\nu(0){\rm e}^{-\tau_\nu}+B_\nu(T)(1-{\rm e}^{-\tau_\nu})
The structure of a star is described by the following equations:
\begin{aligned} \frac{dM(r)}{dr}&=&4\pi\varrho(r)r^2\\ \frac{dp(r)}{dr}&=&-\frac{GM(r)\varrho(r)}{r^2}\\ \frac{L(r)}{dr}&=&4\pi\varrho(r)\varepsilon(r)r^2\\ \left(\frac{dT(r)}{dr}\right)_{\rm rad}&=&-\frac{3}{4}\frac{L(r)}{4\pi r^2}\frac{\kappa(r)}{4\sigma T^3(r)}~,~~\mbox{(Eddington), or}\\ \left(\frac{dT(r)}{dr}\right)_{\rm conv}&=&\frac{T(r)}{p(r)}\frac{\gamma-1}{\gamma}\frac{dp(r)}{dr}~,~~\mbox{(convective energy transport)}\end{aligned}
Further, for stars of the solar type, the composing plasma can be described as an ideal gas:
p(r)=\frac{\varrho(r)kT(r)}{\mu m_{\rm H}}
where \mu is the average molecular mass, usually well approximated by:
\mu=\frac{\varrho}{nm_{\rm H}}=\frac{1}{2X+\frac{3}{4}Y+\mbox{$\frac{1}{2}$}Z}
where X is the mass fraction of H, Y the mass fraction of He and Z the mass fraction of the other elements. Further :
\kappa(r)=f(\varrho(r),T(r),\mbox{composition})~~\mbox{and}~~ \varepsilon(r)=g(\varrho(r),T(r),\mbox{composition})
Convection will occur when the star meets the Schwartzschild criterium:
\left(\frac{dT}{dr}\right)_{\rm conv}<\left(\frac{dT}{dr}\right)_{\rm rad}
Otherwise the energy transfer takes place by radiation. For stars in quasi-hydrostatic equilibrium the approximations r=R, M(r)=M, dM/dr=M/R, \kappa\sim\varrho and \varepsilon\sim\varrho T^\mu hold (this last assumption is only valid for stars on the main sequence). For pp-chains holds \mu\approx5 and for the CNO chains holds \mu=12 tot 18. It can be derived that L\sim M^3: the mass-brightness relation. Further: L\sim R^4\sim T^8_{\rm eff}. This results in the equation for the main sequence in the Hertzsprung-Russell diagram:
^{10}\log(L)=8\cdot^{10}\log(T_{\rm eff})+\mbox{constant}
The net reaction from which most stars gain their energy is: \rm 4{}^1H\rightarrow{}^4He+2e^++2\nu_{\rm e}+\gamma.
This reaction produces 26.72 MeV. Two reaction chains are responsible for this reaction. The slowest, speed-limiting reaction is shown in boldface. The energy between brackets is the energy carried away by the neutrino.
Both ^7Be chains become more important with raising T.
| \longrightarrow | \searrow | |||
| \nearrow | \rightarrow | \rm~^{15}N+p^+\rightarrow\alpha+^{12}C | \rm^{15}N+p^+\rightarrow~^{16}O+\gamma | |
| \downarrow | \downarrow | |||
| \rm^{15}O+e^+\rightarrow~^{15}N+\overline{\nu} | \rm^{12}C+p^+\rightarrow{}^{13}N+\gamma | \rm^{16}O+p^+\rightarrow~^{17}F+\gamma | ||
| \uparrow | \downarrow | \downarrow | ||
| \bf ^{14}N+p^+\rightarrow~^{15}O+\gamma | \rm^{13}N\rightarrow~^{13}C+e^++\nu | \rm^{17}F\rightarrow~^{17}O+e^++\nu | ||
| \downarrow | \downarrow | |||
| \nwarrow | \leftarrow | \rm ^{13}C+p^+\rightarrow{}^{14}N+\gamma | \rm^{17}O+p^+\rightarrow\alpha+{}^{14}N | |
| \longleftarrow | \swarrow | |||